
Designing an Integrated Course
.png)
.png)






Learning Environment & Situational Factors to Consider
.png)
.png)

1. Specific Context of the Teaching/Learning Situation
How many students are in the class? Is the course primary, secondary, undergraduate, or graduate level? How long and frequent are the class meetings? How will the course be delivered: live, online, blended, flipped, or in a classroom or lab? What physical elements of the learning environment will affect the class? What technology, networking, and access issues will influence the class?
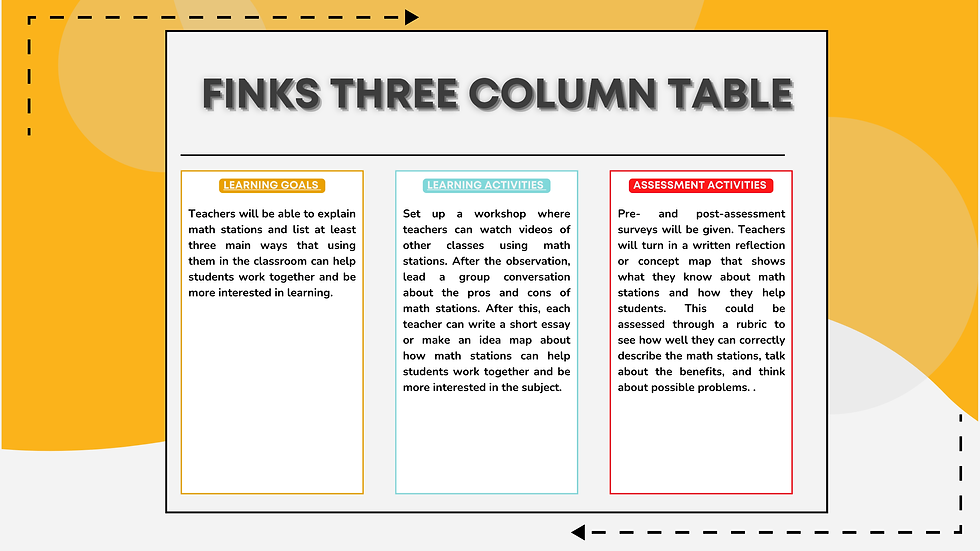
The small class size will promote active participation and personalized learning experiences, with 25 and 30 adult learners per session.
-
Professional learning experiences will be designed as a series of workshops, possibly 2-3 hours each, spread over a school year to allow for implementation and reflection.
-
The course is designed for adult learners and professionals seeking to enhance their skills and knowledge.
-
The course will be delivered through a blended approach, combining online learning modules with in-person workshops and networking events.
-
A blended delivery model could be most effective, combining live workshops (for hands-on activities and collaboration) with online resources and discussions to support learning and allow flexibility.
-
The physical learning environment will be arranged to model math stations, allowing participants to experience and practice setting up various stations.
-
Access to reliable technology is essential, as resources, templates, and collaborative tools will likely be digital. Overcoming networking and access issues will be a priority to ensure all participants can benefit from the course materials.
2. General Context of the Learning Situation
What learning expectations are placed on this course or curriculum by the school, district, university, college, and/or department? the profession? society?
-
The course is expected to align with the Fort Bend Independent School District's professional development goals and the evolving needs of the education industry and society in promoting innovation, collaboration, and lifelong learning.
-
The course will align with the learning expectations from the district or school's curriculum guidelines, professional teaching standards, and best practices in mathematics education.
-
The profession expects teachers to continuously update their instructional methods to cater to diverse learning needs, and society increasingly values STEM education and skills.
-
The course content and outcomes must align with these expectations while offering flexibility to adapt to different school and district goals.
3. Nature of the Subject
Is this subject primarily theoretical, practical, or a combination? Is the subject primarily convergent or divergent? Are there important changes or controversies occurring within the field?
The subject combines theoretical and practical aspects to bridge the gap between traditional knowledge acquisition and real-world application through hands-on activities and digital tools. The subject involves both convergent and divergent thinking, challenging learners to analyze existing practices critically while exploring creative solutions to current challenges in the educational field.
-
Implementing math stations and teaching math developmentally is highly practical, requiring theoretical understanding and hands-on application.
-
It combines convergent (proven strategies that work) and divergent (creativity in implementation based on class needs).
-
The field of math education is dynamic, with ongoing discussions about best practices in teaching math conceptually rather than procedurally; thus, the course content might address these trends and debates.
4. Characteristics of the Learners
What is the life situation of the learners (e.g., socio-economic, cultural, personal, family, professional goals)? What prior knowledge, experiences, and initial feelings do students usually have about this subject? What are their learning goals and expectations?
Life Situation: Learners come from diverse socioeconomic backgrounds, industries, and cultural contexts. They share a commitment to professional growth and advancement.
Prior Knowledge: Teachers typically have foundational knowledge and experience in the subject area but seek to deepen their understanding and acquire new skills to stay competitive in the evolving landscape. Prior knowledge and experience with math stations will also vary, so the course must accommodate a range of starting points, ensuring that every participant can grow and learn effectively.
Learning Goals: Their goals include enhancing job performance, exploring new career opportunities, and engaging in a collaborative learning community to expand their professional network. Teachers may also want to use math stations to improve student engagement, differentiate instruction, and meet curriculum standards.
5. Characteristics of the Teacher
What beliefs and values does the teacher have about teaching and learning? What is his/her attitude toward the subject? students? What level of knowledge or familiarity does s/he have with this subject? What are his/her strengths in teaching?
-
The instructor of this course must believe in a constructivist approach to teaching and learning, valuing the development of understanding over rote memorization.
-
The instructor should have a genuine interest in mathematics and an enthusiasm for innovative teaching methods.
-
Ideally, they will have substantial experience with math stations and developmental teaching in their practice and will be able to share personal insights and experiences.
-
Strengths may include effective communication, the ability to model and deconstruct complex processes, and the capacity to inspire and support other educators in trying new strategies.

Questions for Formulating Significant Learning Goals
.png)
.png)
.png)
I want and hope that teachers will have engaged in meaningful learning experiences a year (or more) after this course is over.
Goal 1: Enhance the participants' abilities and understanding in crucial areas identified during the needs assessment process.
Goal 2: Have confidently integrated math stations into their daily teaching routines, adapting them to different mathematical concepts and student needs.
Goal 3: Continue developing and sharing new math station strategies that foster developmental learning.
Goal 4: Reflect on and assess the effectiveness of math stations in their classrooms, making data-driven adjustments to improve student engagement and learning.
My Big Hairy Audacious Goal (BHAG) for the course is to revolutionize how math is taught in classrooms by establishing math stations as a standard teaching practice and creating an engaging, inclusive, and developmentally appropriate learning environment for all students.
Foundational Knowledge
-
What critical information (e.g., facts, terms, formulae, concepts, principles, relationships, etc.) is/are essential for students to understand and remember in the future?
-
What key ideas (or perspectives) are essential for students to understand in this course?
Essential information includes understanding the principles of developmental learning, the structure of math stations, and how to differentiate instruction within this framework.
Teachers need to understand the pedagogical theories behind math stations, such as constructivism, and the research supporting developmental learning in mathematics.
1. Principles of Developmental Learning: Educators need to understand the ideas behind developmental learning so they can adapt their lessons to meet the needs of their students' mental, social, emotional, and physical growth. The concept behind developmental learning is that students develop at predictable stages, and for learning to be successful, it should be matched to these stages.
2. Structure of Math Stations: Math stations are structured learning environments where students rotate through various activities to practice and reinforce mathematical concepts. Educators must understand how to effectively set up and manage math stations to create a conducive learning environment that promotes active engagement and differentiated instruction.
3. Differentiation within the Framework: Differentiation is the practice of modifying instruction to meet students' needs. Within the framework of math stations, teachers need to be able to differentiate instruction based on students' readiness, interests, and learning profiles to ensure that all students are appropriately challenged and supported in their mathematical learning.
4. Pedagogical Theories Behind Math Stations: Educators should be familiar with pedagogical theories that support the effectiveness of math stations, such as constructivism. Constructivism emphasizes the active role of learners in constructing their understanding of concepts through hands-on experiences, collaboration, and reflection. Understanding these theories can help teachers design and implement math stations that facilitate meaningful learning experiences for students.
5. Research Supporting Developmental Learning in Mathematics: Knowing about the research that supports developmental learning in math can help teachers make smart choices about how to teach and what methods to use. Research in this area shows how to teach math ideas effectively at different stages of development and how important it is to consider what students already know and have done while they are learning.
By grasping these key ideas and essential information, educators can enhance their teaching practices, support student learning effectively, and create engaging and enriching learning experiences in the context of math stations and developmental learning in mathematics.
Application Goals
-
What kinds of thinking are essential for students to learn?
-
Critical thinking, in which students analyze and evaluate
-
Creative thinking, in which students imagine and create
-
Practical thinking, in which students solve problems and make decisions
-
What essential skills do students need to gain?
-
Do students need to learn how to manage complex projects?
1. Critical Thinking:
Critical thinking involves objectively analyzing and evaluating an issue or situation to form a judgment. Critical thinking is essential for students to learn in an educational setting because it enables them to assess the validity of information, arguments, and ideas. It empowers students to discern between fact and opinion, recognize biases, and understand the logical connections between ideas. For instance, in the context of evaluating different math station strategies, students should be able to:
- Assess the strengths and weaknesses of each strategy.
- Understand the underlying principles of the strategies.
- Evaluate the impact of each strategy on their learning.
- Draw conclusions based on evidence and reason rather than on emotion or anecdote.
- Reflect on their own thinking processes and biases that may affect their judgments.
2. Creative Thinking:
Creative thinking is the ability to think in new and original ways to create something novel or to find unique solutions to problems. In education, fostering creative thinking is critical as it encourages students to step beyond conventional boundaries and explore different perspectives. When teachers design unique math station activities tailored to their student's needs, they are promoting creative thinking by:
- Encouraging students to approach problems in innovative ways.
- Allowing students to express their understanding of mathematical concepts artistically or unconventionally.
- Providing opportunities for students to collaborate and share diverse ideas.
- Enabling students to make connections between seemingly unrelated concepts.
- Cultivating an environment where taking intellectual risks is valued.
3. Practical Thinking:
Practical thinking, often called problem-solving, is the ability to handle everyday tasks and troubleshoot issues effectively. It includes decision-making skills and the application of knowledge to real-world situations. Practical thinking is crucial for students to learn as it enables them to:
-
Apply mathematical concepts to solve real-life problems.
-
Use logic and reasoning to identify potential issues while implementing math stations.
-
Make informed decisions about adapting math stations to meet learning objectives and student needs better.
-
Develop strategies for overcoming obstacles and making the most efficient use of resources.
-
Reflect on the outcomes of their decisions to inform future practice.
4. Essential Skills:
To succeed in a dynamic educational environment, students need to gain various skills, including:
-
Classroom management within a math station framework, ensuring that all students are engaged and resources are used effectively.
-
Designing math station activities that align with educational standards and learning objectives.
-
Assessing the effectiveness of math station activities and student performance.
-
Collaborating with peers to share best practices and improve instructional methods.
-
Communicating effectively with students to provide clear instructions and constructive feedback.
5. Complex Project Management:
Managing complex projects is an invaluable skill for students, preparing them for future academic and professional endeavors. In the context of math stations, students, as well as teachers, may need to:
-
Plan and organize multiple activities, ensuring that each station runs smoothly and contributes to the overall learning goals.
-
Coordinate with other educators to integrate math stations into the broader curriculum, ensuring coherence and continuity in learning.
-
Manage time and resources efficiently to maximize educational outcomes.
-
Monitor progress and adapt plans as necessary based on formative assessments and student feedback.
-
Reflect on the project management process to identify areas for improvement and apply lessons learned to future projects.
Integration Goals
-
What connections (similarities and interactions) should students recognize and make…:
-
Among ideas within this course?
-
Among the course's information, ideas, and perspectives and those in other classes or areas?
-
Among the material in this course and the student's personal, social, and work life?
Students should recognize connections between math station activities and broader mathematical concepts.
They should integrate ideas from this course with other educational strategies and subjects they teach.
The course should encourage teachers to relate the math station approach to their personal teaching philosophy and their students' diverse learning styles.
1. Connections Among Ideas Within the Course:
Students should be able to identify and synthesize the underlying principles that connect various topics within the course itself. For instance, when dealing with mathematical concepts such as algebra, geometry, and statistics, students should be encouraged to understand how these areas are not isolated islands of knowledge but interconnected systems that share common logic and problem-solving techniques. They should recognize that skills developed in one area can enhance understanding in another. For example, algebraic manipulations might be crucial in solving geometric proofs, or statistical methods may be applied to interpret algebraic functions.
Furthermore, students should be encouraged to draw parallels between mathematical models and theorems, understanding how a principle learned in one context can be abstracted and applied to another. This could involve recognizing patterns and structures that recur within the course content, which can lead to a deeper comprehension of the subject as a whole.
2. Connections Between This Course and Other Courses or Areas:
Students should be encouraged to make interdisciplinary connections and understand how the ideas and methods from their math course can be relevant and applied to other subjects they are studying. For instance, the precision and logical reasoning skills honed in mathematics are vital in scientific inquiry. Mathematical modeling is essential in economics for understanding markets and computer science for developing algorithms.
Moreover, the course should highlight how mathematical literacy is valuable for critical thinking and problem-solving in various disciplines, from the arts to the social sciences. For example, in history or social studies, students might use statistical data to analyze trends, while in art, they may explore geometric concepts in design. This approach enriches their learning experience and prepares them for a more integrated view of knowledge, promoting lifelong learning skills.
3. Connections Between Course Material and Personal, Social, and Work-Life:
The course should encourage students to see the relevance of mathematics to their everyday lives, emphasizing how mathematical thinking can enhance personal decision-making, such as budgeting or planning projects. It should also be clear how mathematics plays a role in social understanding, such as interpreting graphs and statistics presented in media or understanding demographic data that can inform social policy decisions.
In terms of work life, students should be able to identify how the skills and knowledge they acquire from math stations and the course at large can be applied to various professional contexts. Data analysis and quantitative reasoning are indispensable for many careers, from engineering to marketing. Teachers should be equipped to illustrate these connections, making mathematics an academic requirement and a practical tool that students can wield in their future careers.
Through these integration goals, the course aims to create a cohesive learning experience that not only builds mathematical competence but also fosters an appreciation for the utility and beauty of mathematics in the broader tapestry of students' lives and the world around them.
Human Dimensions Goals
-
What could or should students learn about themselves?
-
What could or should students learn about understanding others and interacting with them?
Teachers should learn about their teaching style and how to be adaptive and responsive educators.
They should learn about the diverse needs of learners in their classrooms and how to foster an inclusive environment using math stations.
1. What could or should students learn about themselves?
Students, the teachers, should self-reflect to understand their learning styles, strengths, weaknesses, and interests. By gaining insight into their unique characteristics, preferences, and abilities,they can develop effective learning strategies and set meaningful goals for their academic and personal growth. This self-awareness can empower students to take ownership of their learning journey, make informed decisions, and cultivate a positive self-concept. Students should use reflective practices to evaluate their instructional methods, classroom management strategies, and communication approaches. By understanding their teaching styles, strengths, and areas for improvement, educators can tailor their teaching practices to meet students' diverse needs and learning preferences. Adaptive and responsive educators involve being open to feedback, experimenting with innovative teaching techniques, and continuously refining their pedagogical skills to create engaging and effective learning experiences for all learners.
2. What could or should students learn about understanding others and interacting with them?
Students should develop empathy, communication skills, and cultural competence to enhance their ability to understand and collaborate with diverse individuals. By recognizing and respecting the perspectives, backgrounds, and experiences of others, students can build meaningful relationships, resolve conflicts constructively, and contribute positively to a collaborative learning community. Through interactions with peers and educators, students can cultivate social skills, teamwork, and leadership qualities essential for academic, professional, and social success.They should learn about the diverse needs of learners in their classrooms and how to foster an inclusive environment using math stations. Teachers should learn about students' diverse learning profiles, interests, abilities, and backgrounds to design inclusive and differentiated instruction. By implementing strategies such as math stations, teachers can provide students with opportunities for personalized learning, hands-on exploration, and collaborative problem-solving. Creating an inclusive environment entails promoting equity, diversity, and accessibility in the classroom so all students feel valued, supported, and empowered to thrive academically and socially.
Caring Goals
-
What changes/values do you hope students will adopt?
Feelings?
Interests?
Values?
I hope teachers adopt a value for student-centered learning and develop a passion for teaching math in an engaging and developmentally appropriate way. Teachers should be genuinely interested in continuing to evolve and improve their teaching practices. It is my dream for this course to foster a caring attitude toward students' mathematical journeys and the belief that every child can succeed in math. When discussing the changes and values I hope students will adopt, I envision a multi-dimensional transformation that extends to their emotional, intellectual, and ethical development.
Feelings:
I aim for students to experience a positive shift in their emotional engagement with learning. They must develop confidence in their abilities, which can be achieved through a supportive classroom environment that acknowledges and celebrates their progress. By cultivating an atmosphere where mistakes are viewed as learning opportunities rather than failures, I expect students to foster a resilience that allows them to tackle challenges with perseverance and optimism. The ultimate goal is for students to associate positive feelings with their educational journey, thus creating a self-sustaining cycle of motivation and enthusiasm for learning.
Interests:
In terms of interests, students will become more curious about the world around them. By incorporating real-world applications and interdisciplinary connections into the curriculum, students should develop a keen interest in understanding how mathematical concepts apply outside the classroom. This approach makes math more relatable and sparks curiosity in other subjects and areas of life, fostering lifelong learners eager to explore and understand new concepts and ideas.
Values:
From a values perspective, I expect students to adopt a strong sense of integrity and responsibility in their learning. This includes the value of hard work, the importance of honesty in their academic endeavors, and a deep respect for the collaborative learning process. I want to instill in them the value of critical thinking and the ability to approach problems with formulaic solutions, creativity, and ethical consideration.
Teacher Development:
Moving on to teacher development, I aspire for teachers to embrace a student-centered approach to teaching math wholeheartedly. This involves recognizing and catering to the diverse learning needs of each student and facilitating a classroom that is responsive and adaptive to those needs. I envision teachers developing an infectious enthusiasm for math, igniting students' passion. By showcasing math as a dynamic and exciting discipline, teachers can create a learning environment where students look forward to engaging with mathematical concepts.
Continuous professional development is another crucial aspect for teachers. I hope they will pursue a sustained interest in reflecting upon and enhancing their teaching methodologies. This includes staying abreast of the latest educational research, integrating technology effectively into the classroom, and participating in professional learning communities. By committing to lifelong learning, teachers can model the growth mindset they wish to instill in their students.
Caring Attitude and Belief in Success:
Lastly, the course should encourage a nurturing and empathetic approach to each student's mathematical journey. Teachers should foster a caring attitude sensitive to students' emotional and academic needs, empathizing with their struggles and celebrating their triumphs. Teachers need to be convinced that every child, regardless of their starting point, can succeed in math. By embracing this belief, teachers can create an inclusive and encouraging environment that motivates all students to strive for excellence and reach their full potential in math and beyond.
"Learning-How-to-Learn" Goals
-
What would you like for students to learn about:
-
How can we be good students in a course like this?
-
How to learn about this particular subject?
-
How do you become a self-directed learner of this subject, i.e., have a learning agenda of what you need/want to learn and a plan for learning it?
Teachers should learn how to be proactive in seeking out new math station ideas and resources.
They should learn to critically assess their teaching's impact on students' learning and development in math.
I would like them to become self-directed learners who continually seek to improve their understanding and implementation of math stations, setting goals for their professional development in this area.
These goals aim to create a comprehensive learning experience for teachers that imparts knowledge and skills and inspires a long-term commitment to developmentally appropriate math instruction.
For Their Students:
1. Becoming Effective Students:
I wish for the educators who take my course(s) to foster an environment in their classrooms where they encourage their students to internalize the qualities and habits of good students. This includes developing effective study strategies, time management skills, and critically evaluating their understanding. They should learn to ask insightful questions, participate actively in class discussions, and collaborate effectively with peers. Encouraging them to reflect on their learning processes and outcomes is equally important, as this reflection can lead to better self-awareness and adjustments in their approach to studying.
2. Learning About the Subject:
I aim for educators to encourage their students to engage deeply with the subject, moving beyond rote memorization to profound comprehension. This involves understanding key concepts, applying them to various contexts, and developing problem-solving skills appropriate to the subject. Students should also learn to connect new information with their knowledge, constructing a well-organized framework that facilitates retention and recall.
3. Becoming Self-Directed Learners:
I envision teachers facilitating students taking ownership of their learning journey. This means they must identify what they need and want to learn, set clear goals, and develop actionable plans to achieve them. It includes the ability to self-assess proficiency, recognize areas for improvement, and seek out resources and opportunities to learn independently. A self-directed learner is curious, motivated, and resilient, capable of adapting to new challenges and learning environments.
For Teachers:
1. Proactivity in Resource Gathering:
Teachers should actively seek out inspiration or resources, not passively wait for inspiration or resources. This means exploring a variety of sources for creative math station ideas, engaging with professional learning communities, and staying abreast of current research and best practices in mathematics education.
2. Critical Assessment of Teaching Impact:
I want teachers to be reflective practitioners who critically assess the effectiveness of their teaching strategies on students' learning outcomes. This involves regularly analyzing student work, seeking feedback, and observing student engagement and understanding during lessons. Teachers should be willing to adjust their methods and experiment with new approaches to maximize learning.
3. Becoming Self-Directed Professionals:
Encouraging teachers to be lifelong learners in math education is a crucial goal. This includes setting personal professional development goals, pursuing further education, attending workshops and conferences, and staying informed about emerging educational technologies and methodologies. Teachers should be self-directed learning models, demonstrating a commitment to continuous improvement and excellence in their craft.
By setting these "Learning-How-to-Learn" goals, I aim to develop an environment where both students and teachers are not just recipients of knowledge but active participants in the learning process. The overarching aim is to foster an educational culture that values growth, adaptability, and the pursuit of excellence, specifically focusing on effective mathematics teaching and learning through dynamic and engaging math stations.

References
Diller, D. (2011, January 1). Math Work Stations. Stenhouse Publishers. http://books.google.ie/books?id=CVMThu5IndYC&pg=PA69&dq=9781571107930&hl=&cd=1&source=gbs_api
Fink, L. Dee, PhD. A Self-Directed Guide to Designing Courses for Significant Learning. N.p.: n.p., n.d. PDF.
Van De Walle, J. A., Karp, K., & Bay-Williams, J. M. (2010, January 1). Elementary and Middle School Mathematics. http://books.google.ie/books?id=w_zuAAAAMAAJ&q=9780134802084&dq=9780134802084&hl=&cd=1&source=gbs_api